Acceleration: Definition, Formula, Types, Problems & Examples

A non-uniform motion is one in which the velocity of the particle changes. The velocity may change due to a change in magnitude(speed), a change in the direction of motion, or both. In one dimensional motion, the motion takes place in a specified direction and the change in velocity occurs due to a change in the magnitude of velocity alone.

Contents

1 Acceleration – Definition
2 Acceleration – Formula
3 Types of acceleration
4 Acceleration due to gravity
5 General relations connecting Position, Velocity and Acceleration
6 Application of Differentiation and Integration in uniformly accelerated motion
- 6.1 Velocity – time relation
- 6.2 Displacement – time relation
7 Acceleration Problems & Solutions

Acceleration – Definition

When the velocity of an object changes with time, it is said to have an acceleration. Thus, the acceleration is defined as the rate of change of velocity of a body. $large begin{aligned}i.e.,; acceleration =frac{change;in;velocity}{time} end{aligned}$

The SI unit of acceleration is ms^-2and its dimensional formula is [M⁰L¹T^-2].

The acceleration is a vector quantity since the velocity is a vector quantity. However, the direction of motion of a body is given by the direction of velocity and not by the direction of acceleration. For example, in the case of a body going up, the velocity is directed upwards while its acceleration is in the downward direction.

The acceleration may be either positive, negative or zero. When the velocity of the body is increasing with time, acceleration has a positive value and the body is said to be in accelerated motion. On the other hand, if the velocity of the body is decreasing with time, the acceleration is negative and the body is said to be in decelerated or retarded motion. Negative acceleration is called deceleration or retardation. If the body moves with uniform velocity, its acceleration is zero.

Example: The velocity of a body falling body does not change its direction but its magnitude changes uniformly with time. Therefore the body has an acceleration.

Acceleration – Formula

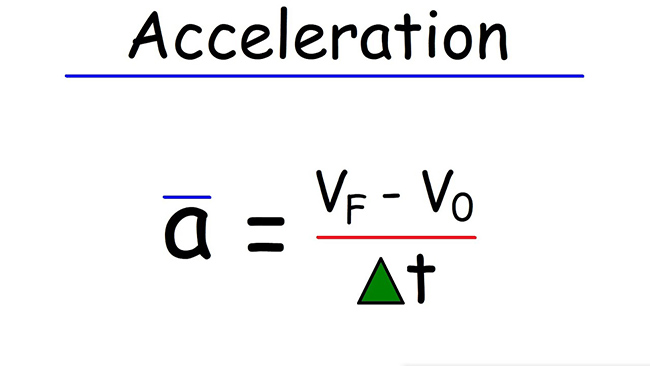
If the acceleration at every instant of motion is the same, the object is said to move with a uniform or constant acceleration.

Consider an object moving with a uniform acceleration ‘a’. If v₁ and v₂ are its velocities at the instants t₁ and t₂ respectively (where t₂ > t₁), then the acceleration of the object is given by, $large begin{aligned} acceleration,;a =frac{v_{2}-v_{1}}{t_{2}-t_{1}} end{aligned}$

If the velocity v₂ > v₁, that is, the velcoity is increasing during the interval, the acceleration is said to be positive.
If the velocity v₂ < v₁, that is, the velocity is decreasing with time, the acceleration is negative and is called deceleration or retardation.

That is, in general terms we can say, acceleration means speeding up while retardation means slowing down.

Similarly, for a particle having velocity v₀ at t = 0 and velocity v_t after a time t, the acceleration is given by, $large begin{aligned} a =frac{v_{t}-v_{0}}{t} end{aligned}$

Types of acceleration

In general we can classify acceleration as uniform acceleration, average acceleration and instantaneous acceleration.

Uniform Acceleration:

As said earlier, a body is said to be moving with uniform acceleration, if equal changes in velocity take place in equal intervals of time, however small these intervals be.
Example: motion of a freely falling body

Average Acceleration:

If the acceleration of a particle is not uniform over a time interval, the particle is said to move with non uniform acceleration. The ratio of change in velocity to the time is called average acceleration. $large begin{aligned} i.e., Average;acceleration,; a_{av} =frac{change;in;velocity}{time} end{aligned}$

Let (v₂ – v₁) be the change in velocity in an interval of time (t₂ – t₁). Then, the average acceleration is given by, $large begin{aligned} Average;acceleration,; a_{av} =frac{v_{2}-v_{1}}{t_{2} -t_{1}} end{aligned}$

Instantaneous Acceleration:

The acceleration of an object at any instant is called instantaneous acceleration.

Let Δv is the change in velocity in an infinitely small interval of time Δt, around t, then the instantaneous acceleration a_t at the instant t is given by, $large begin{aligned} i.e., a_{t} =lim_{Delta t to 0}frac{Delta v}{Delta t}&=frac{dv}{dt} \but;we;&know,;v=frac{dx}{dt} \therefore a_{t} &=frac{d}{dt}left ( frac{dx}{dt} right ) \&=frac{d^{2}x}{dt^{2}} end{aligned}$

That is, the instantaneous acceleration is the second derivative of position with respect to time.

Acceleration due to gravity

The earth attracts all bodies towards its centre. Hence if an object is dropped from a height, it falls freely downwards with uniform acceleration. This uniform acceleration is called acceleration due to gravity and is denoted by ‘g’. The value of ‘g’ is nearly 9.8 ms^-2 for all freely falling bodies near the surface of the earth.

The value of ‘g’ varies from place to place on the surface of the earth. Its value is maximum at the poles and minimum at the equator. Above and below the surface of the earth, its value is less than that on the surface of the earth. The value of g is zero at the centre of the earth.

General relations connecting Position, Velocity and Acceleration

The general relations connecting position co-ordinate and velocity in one dimensional motion is given by, $large begin{aligned} v =lim_{Delta t to 0}frac{Delta x}{Delta t}&=frac{dx}{dt} end{aligned}$

Thus velocity of an object is the derivative of position with respect to time.

Similarly, the relation connecting the velocity of an object at the instant t and the acceleration of the object is given by, $large begin{aligned} a_{t} =lim_{Delta t to 0}frac{Delta v}{Delta t}&=frac{dv}{dt} \but;v=frac{dx}{dt} \therefore a &=frac{d}{dt}left ( frac{dx}{dt} right ) \&=frac{d^{2}x}{dt^{2}} end{aligned}$

Thus acceleration of an object is the second derivative of position with respect to time.

Application of Differentiation and Integration in uniformly accelerated motion

Velocity – time relation

The instantaneous accelertion of an object in uniformly accelerated motion is given by, $large begin{aligned} a&=frac{dv}{dt} \therefore dv &=adt;;;;---------(1) end{aligned}$

Let u be the velocity of the object at time t = 0 and v be the velocity at time t.
Integrating both sides of equation (1) within the limits of initial velocity u and final velcoity v and time t = 0 and t = t, $large begin{aligned} int_{u}^{v}dv&=int_{0}^{t}adt \&=aint_{0}^{t}dt;;;;;(object;moves;with;uniform;acceleration,therefore;a;is;constant) \therefore;;;left [ v right]_{u}^{v}&=aleft [ t right ]_{0}^{t} \ie.,;;v-u&=aleft [ t-0 right ] \ie.,;;v-u&=at \or,;;; v&=u+at;;;;;-------(2) end{aligned}$

Displacement – time relation

The instantaneous velocity of an object when it is displaced through ds is given by, $large begin{aligned} v&=frac{ds}{dt} \therefore;;;ds&=vdt end{aligned}$

As obtained in the previous section {equation (2)}, if the initial velocity of the object at time t = 0 be u, and the velocity at time t = t be v, then $large begin{aligned} v&=u+at \ie.,;;frac{ds}{dt}&=u+at \therefore;;; ds&=(u+at)dt \or,;;;ds&=u;dt+at;dt;;;;;--------(3) end{aligned}$

Integrating both sides of the above equation between limits s = 0 to s and time t = 0 to t, we get, $large begin{aligned} int_{0}^{s}ds&=int_{0}^{t}udt+int_{0}^{t}atdt \&=uint_{0}^{t}dt+aint_{0}^{t}tdt;;;;;because a;and;u;are;constants \therefore;;;left [ s right]_{0}^{s}&=uleft [ t right ]_{0}^{t}+aleft [ frac{t^{2}}{2} right ]_{0}^{t} \ie.,;;s&=ut+frac{1}{2}at^{2};;;;;-------(4) \Again;on;&differentiating;equation;(4);with;respect;to;t, \ie.,;;;;frac{ds}{dt}&=frac{d}{dt}(ut)+frac{d}{dt}(frac{1}{2}at^{2}) \ie.,;;;;v&=u+frac{1}{2}a.2t \we;get,;;;;v&=u+at;;;;;--------(5) end{aligned}$

Acceleration Problems & Solutions

Problem 1:
The displacement of a particle moving along a straight line is x = k₀ + k₁t + k₂t² where k₀, k₁ and k₂ are constants. Find the acceleration.

Answer:
$large begin{aligned} Given,;Displacement,;x&=k_{0}+k_{1}t+k_{2}t^{2} \Velocity,;v&=frac{dx}{dt} \&=frac{d}{dt}(k_{0}+k_{1}t+k_{2}t^{2}) \&=k_{0}.0+k_{1}.1+k_{2}.2t \&=k_{1}+2k_{2}t \Acceleration,;a&=frac{dv}{dt} \&=frac{d}{dt}(k_{1}+2k_{2}t) \&=k_{1}.0+2k_{2}.1 \&=2k_{2} end{aligned}$

Problem 2:
The position of an object at any time t is given by the relation x = mt + nt² + pt³, where m, n and p are constants. Find the instantaneous acceleration of the object at t = 8 seconds.

Answer:
$large begin{aligned} Given,;Position;of;the;object,;x&=mt+nt^{2}+pt^{3} \Velocity,;v&=frac{dx}{dt} \&=frac{d}{dt}(mt+nt^{2}+pt^{3}) \&=m.1+n.2t+p.3t^{2} \&=m+2nt+3pt^{2} \Acceleration,;a&=frac{dv}{dt} \&=frac{d}{dt}(m+2nt+3pt^{2}) \&=m.0+2n.1+3p.2t \&=2n+6pt \At;time;t=8s,;&the;instantaneous;acceleration, \a_{ins}&=a_{t=8} \&=2n+6ptimes 8 \&=2n+48p end{aligned}$

Problem 3:
The distance x of a particle moving in one dimension, under the action of a constant force, is related to time t by equation $large begin{aligned} t=sqrt{x}+6 end{aligned}$ where x is in metre and tin seconds. Find the displacement of the particle when (a) its velocity is zero (b) its velocity is 4 m/s.

Solution:
$large begin{aligned} Given,;t&=sqrt{x}+6 \sqrt{x}&=t-6 \Squaring;both;sides,x&=(t-6)^{2} \&=t^{2}-12t+36 \therefore velocity,;;v&=frac{dx}{dt} \&=frac{d}{dt}(t^{2}-12t+36) \&=2t-12 \\(a);;When;velocity,;v=0, \0&=2t-12 \ie.,;;;2t&=12 \therefore;;;;;t&=frac{12}{2} \&=6;seconds \At;t=6sec,;;Dis&placement,;x=t^{2}-12t+36 \&hspace{2.89cm}=6^{2}-12times 6+36 \&hspace{2.89cm}=36-72+36 \&hspace{2.89cm}=0 \&hspace{-5.7cm}(b);;When;velocity,;v=4;m/s, \4&=2t-12 \ie.,;;;2t&=12+4 \therefore;;;;;t&=frac{16}{2} \&=8;seconds \At;t=8sec,;;Dis&placement,;x=t^{2}-12t+36 \&hspace{2.89cm}=8^{2}-12times 8+36 \&hspace{2.89cm}=64-96+36 \&hspace{2.89cm}=4;m end{aligned}$

Problem 4:
The acceleration of an object is given by a = α + βt, where α and β are constants. At time t = 0, let x₀ and v₀ are the position and velocity of the object. Find the expression for position and velocity of the object at any time t.

Solution: $large begin{aligned} \Velocity;of;the;object;at;any;time;t;is;; v&=v_{0}+alpha t+frac{1}{2}beta t^{2} \Position;of;the;object;at;any;time;t;is;; x&=x_{0}+v_{0}t+frac{1}{2}alpha t^{2}+frac{1}{6}beta t^{3} end{aligned}$

Problem 5:
The position of an object moving along x axis is given by x = a + bt² where a = 8.5 m, b = 2.5ms^-2 and t is measured in second. What is its velocity at t = 0s and t = 2s? What is the average velocity between t = 2 s and t = 4 s?

Answer: $large begin{aligned} Given,;x&=a+bt^{2} \therefore velocity,;v&=frac{dx}{dt} \&=frac{d}{dt}left ( a+bt^{2} right ) \&=0+b.2t \&=2bt \Given,;b=&2.5;ms^{-2} \therefore velocity;at;t=0;is,;v&=2bt \&=2times 2.5times 0 \&=0;ms^{-1} \Again;velocity;at;t=2;is,;v&=2bt \&=2times 2.5times 2 \&=10;ms^{-1} \Now;we;need;to;find;the;&average;velocity;between;t=2s;and;t=4s.; \Given,;position,;x&=a+bt^{2} where;a=8.5;m;and;b=2.5;ms^{-2} \therefore the;position;of;the;object;at;t=2s;is,;x_{1}&=8.5+2.5times 2^{2} \&=18.5;m \Similarly,;the;position;of;the;object;at;t=4s;is,;x_{2}&=8.5+2.5times 4^{2} \&=48.5;m \therefore;average;velocity;between;t=2s;and;t=4s;is,;V_{avg}&=frac{x_{2}-x_{1}}{t_{2}-t_{1}} \&=frac{48.5-18.5}{4-2} \&=frac{30}{2} \&=15ms^{-1} end{aligned}$

Problem 6:
The displacement of a particle along x axis is given by x = 3 + 8t + 7t². Obtain its velocity and acceleration at t = 2 s.

Solution: $large begin{aligned} Given,;; x&=3+8t+7t^{2} \Then,; velocity,;v&=frac{dx}{dt} \&=frac{d}{dt}(3+8t+7t^{2}) \&=0+8times 1+2times 7times t \&=8+14t \therefore velocity;at;t=2s;is,;V_{t=2s}&=8+14times 2 \&=36;ms^{-1} \Now, acceleration,;a&=frac{dv}{dt} \&=frac{d}{dt}(8+14t) \&=0+14times 1 \&=14;ms^{-1} \i.e.,;acceleration;at;t=2s;is,;a_{t=2s}&=14;ms^{-1} end{aligned}$

I hope the information in this article helps you to get a brief idea about the concept of acceleration. If you believe I missed something or if you have any suggestions, do let me know via comments.

Stay tuned with HelpYouBetter to learn more about the equations of motion with constant acceleration, the concept of relative velocity and other related topics.

Rate this post

Science

What is Acceleration? – Definition, Formula, Types & Solved problems